Statics forces solved problems

▲

According to the second law of motion we have	F = m a
In the gravitational system the force acting on a mass is	W = m g [kgm m / s² ] = 1 N
A force of 1 Newton is equal to	N = 1 [kgm m / s² ] = 0.102 kgf
Then if the weight is given it equals	W [kgf ] = 9.8 N

▲

m		kgm
M		kgm
α		deg
θ		deg
T		N
F_x		N
F_y		N
F		N
γ		deg

A beam AB whose weigh is M and is located at an angle of α from the wall and is hinged at point A to the wall, a cable is connected to the wall and the beam end at point B as shown in the figure. Find the direction and the value of the force acting on hinge A and the tension in the cable CB.

From the free body diagram, we can write the equilibrium equations in the x and y directions:

x direction:	F_x − T sinθ = 0	(1)
y direction:	F_y + T cosθ − mg − M g = 0	(2)

Another condition that should be fulfilled is that the sum of the moments at the hinge A is ΣM_A = 0

If α + θ is not 90 degree then we have to calculate the projection of T that is perpendicular to the beam which is T sin(α + θ)

Moments at A:

m g L sinα + M g sinα L / 2 − T sin(α + θ) L = 0

(3)

from eq. (3) we can find the value of T.

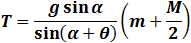	(4)
Substitute eq. (4) into eq. (1) and (2) we get the forces F_x and F_y :
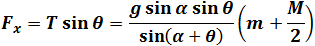	(5)
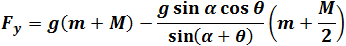	(6)

Once we found F_x and F_y we can find the reaction force F and the angle of the reaction γ on hinge A.

If α + θ = 90 degree then T F_x and F_y are:

The weight of a uniform horizontal beam is 10kgf a weight of
m = 500kgf is hanged on point B . A cable holds the end of the beam to the wall at an angle of 60 degree. Find the reaction force on hinge A and its direction. (run example).

Because α + θ = 150 degree we have to use eq. (4) (5) and (6).

Given the weight W which is equal to m g = W

Tension is:	T = sin 90 (500 + 10 / 2)/cos(90 + 60 − 90) = 1010 kgf
F_x is:	F_x = sin90 sin60 (500 + 10 / 2) / sin150 = 874.7 kgf
F_y is:	F_y = 510 − sin90 cos60 (500 + 10 / 2) / sin150 = 5 kgf
γ is:	γ = arctan (874.7 / 5) = 89.7 degree

▲

m		kgm
α		deg
β		deg
T₁		N
T₂		N

From the free body diagram sum of all forces in the x and y directions should be equal to 0.

x direction	T₁ cosα − T₂ cosβ = 0
y direction	m g − T₁ sinα − T₂ sinβ = 0

Solving for T₁ and T₂ we get:

▲

m		kgm
θ		deg
α		deg
T₁		N
T₂		N

Given the value of the mass and the angles θ and α as shown in the figure. Find the tension in the cables holding the mass.

Sum of all forces in the x and y directions should be equal to 0.

In the x direction:	T₂ cosθ − T₁ cosα = 0	(1)
In the y direction:	m g + T₁ sinα − T₂ sinθ = 0	(2)

From eq. (1) and (2) we can find the values of T₁ and T₂

Note: angle θ should be bigger then angle α (θ > α) otherwise T₁ is

negative, but the cable can hold only positive tension.

When α = 0 (cable is horizontal) the equations of T₁ and T₂ are.

▲

W		kgm
M		kgm
θ		deg
a		m
b		m
T		N
F		N
F_x		N
F_y		N
γ		deg

Beam AB is supported by a cable to the wall and makes an angle of θ with the beam, a weight W [kgf] is suspended from the beam at a distance a from the wall as shown in the figure. Determine the tension T in the supporting cable and the direction and value of the force on the pin at point A.

From equilibrium all forces and moments in x and y directions are 0.

x direction	F_x − T cosθ = 0	(1)
y direction	F_y + T sin θ − W − M = 0	(2)
Moments at A	a W + M (a + b) / 2 − (a + b) T sinθ = 0	(3)

We got three equations with F_x F_y and T as unknowns, solving by matrix form we get.

From eq. (3) we can find the tension in the cable.

And the force angle γ is: