☖ Home ⇄ Units Converter ⧉ Geometry Σ Math ✈ Physics 〶 Electricity

Three tangent circles calculator

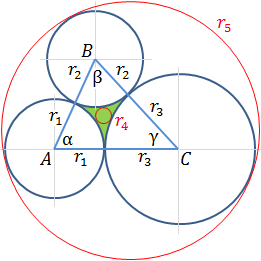
Print 3 tangent circles summary
3 tangent circles
r1
r2
r3
Green area
Green area length
Triangle ABC area
Angle α
Angle β
Angle γ
Inner circle radius   r4
Outer circle radius   r5
              

Area between three tangent circles

Print area between three tangent circles
We define: a = r2 + r3 b = r1 + r3 c = r1 + r2
The area of triangle ABC is:
Triangle ABC area
The sectors area are:
Sectors area
And the area between the 3 tangent circles (green area) is:            A = AT − AA − AB − AC
The angles of the triangle ABC can be found by cosine law:
Triangle angles
The green area circumference is:
P = α r1 + β r2 + γ r3
The radii of the four tangent circles are related to each other according to Descartes circle theorem:
Descartes' theorem
If we define the curvature of the  nth  circle as: Circle curvature
The plus sign means externally tangent circle like circles   r1 , r2 , r3 and r4   and the minus sign is for internally tangent circle like circle   r5 in the drawing in the top.
Then the Descartes circle theorem is:
( k1 + k2 + k3 + k4 ) 2 = 2 ( k12 + k22 + k32 + k42 )
And the curvature of the circles k4 and k5 which are called the Soddy circles are:
Inner circle radius
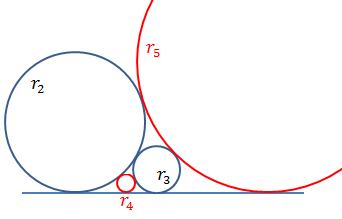Descartes' circle theorem If circle r1 is a straight line then   r1 = ∞
and the curvature is   k1 = 1 / r1 = 0
The curvature of the two red Soddy circles are simply:
Inner circle radius

Area between three tangent circles example

Print area between three tangent circles example
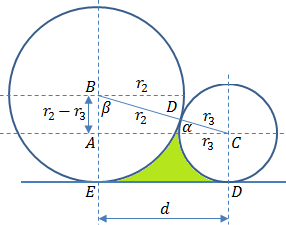Descartes' circle theorem
First, we will find the value of   d
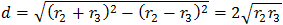
Descartes' circle theorem Descartes' circle theorem
The area of the trapezoid   BCDE   is:
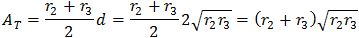
Descartes' circle theorem
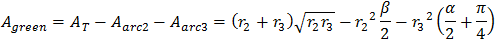
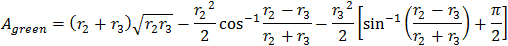
Green area Green area
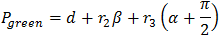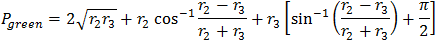
The circumference of the green area is: Circumference
Circumference
NOTE: in all the calculations we assumed that   r2 > r3